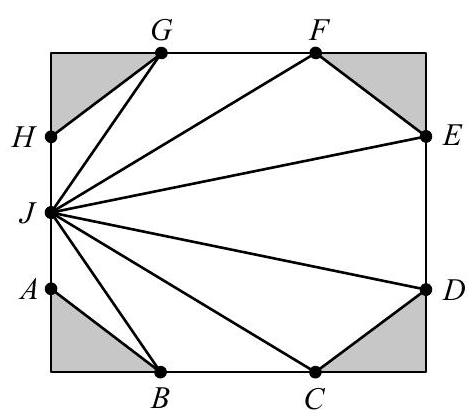
Octagon A B C D E F G H with side lengths A B=C D=E F=G H=10 and B C=D E=F G=H A=11 is formed by removing four 6-8-10 triangles from the corners of a 23 \times 27 rectangle with side \overline{A H} on a short side of the rectangle, as shown. Let J be the midpoint of \overline{H A}, and partition the octagon into 7 triangles by drawing segments \overline{J B}, \overline{J C}, \overline{J D}, \overline{J E}, \overline{J F}, and \overline{J G}. Find the area of the convex polygon whose vertices are the centroids of these 7 triangles.