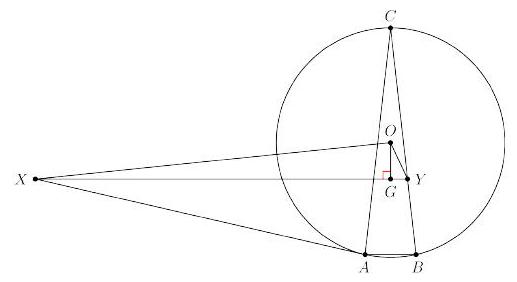
Let \triangle A B C be an acute triangle with circumcenter O and centroid G. Let X be the intersection of the line tangent to the circumcircle of \triangle A B C at A and the line perpendicular to G O at G. Let Y be the intersection of lines X G and B C. Given that the measures of \angle A B C, \angle B C A, and \angle X O Y are in the ratio 13: 2: 17, the degree measure of \angle B A C can be written as \frac{m}{n}, where m and n are relatively prime positive integers. Find m+n.