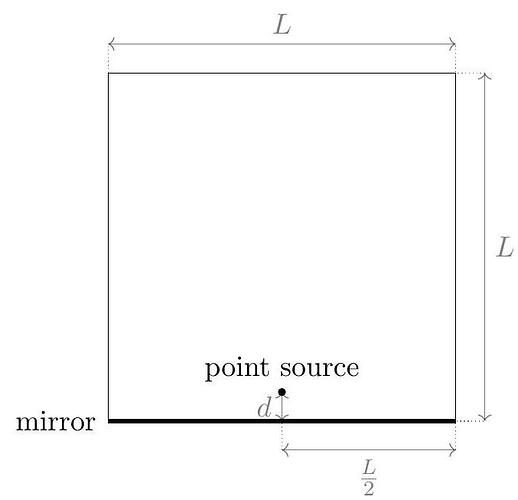
Consider a square room with side length L. The bottom wall of the room is a perfect mirror.* A perfect monochromatic point source with wavelength \lambda is placed a distance d above the center of the mirror, where \lambda \ll d \ll L.
*Remember that the phase of light reflected by a mirror changes by 180^{\circ}.
a. On the right wall, an interference pattern emerges. What is the distance y between the bottom corner and the closest bright fringe above it? Hint: you may assume \lambda \ll y \ll L as well.
b. You plan on running an experiment to determine \lambda in a room with L=40 \mathrm{~m}, and you know that \lambda is between 550 and 750 \mathrm{~nm}. You will measure d and y_{10} (the distance of the tenth fringe from the corner) with the same ruler (with markings of 1 \mathrm{~mm} ). At what d should you place the point source to minimize your error in your \lambda measurement? Roughly what is that minimum error?
c. Now suppose we place a transparent hemispherical shell of thickness s and index of refraction n over the source such that all light from the source that directly strikes the right wall passes through the shell, and all light from the source that strikes the mirror first does not pass through the shell.
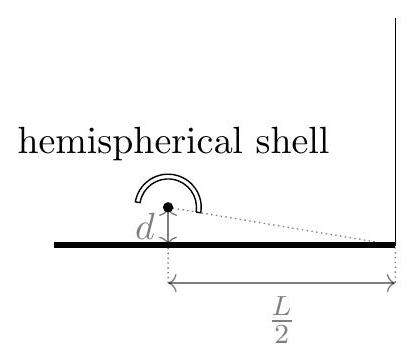
At what y is the fringe closest to the bottom-most corner now? (You may find it convenient to use \lfloor x\rfloor, the largest integer below x.) What is the spacing between the fringes now? Ignore any reflections or diffraction from the hemispherical shell.
d. Now, suppose the hemispherical shell is removed, and we instead observe the interference pattern on the top wall. To the nearest integer, what is the total number of fringes that appear on the top wall? You may assume that d \ll L.